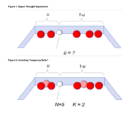
Professor Mannboltz is a world expert on statistical mechanics. A testing service contracted the professor to determine the ranges of scores for the grades on a standardized exam, before the test is administered to many students around the world. The exam is scored from 0 to 2,400 points. There will be 15 grades from highest to lowest: A, B, C, D and F, each with the option to add a + (plus) or – (minus) suffix. The service requests that the resulting distribution of grades among the students to be as close as possible to the following:

The only other information Mannboltz is given is that students always average close to 30% of the possible points on a test like this. Otherwise, he knows absolutely nothing else about the test, the students, etc. What ranges of scores do you think Professor Mannboltz will come up with?

Identity Politics

Here is a meticulously detailed solution to the March-April puzzle, submitted by master puzzle-solver Bob Conger.

Let C denote the population share of Paleomorphs, with 0 < C < 1.

Let D denote the population share of Neomorphs, with 0 < D < 1.

As such, C = 1 – D.

Let P denote the voting parameter as described in the puzzle, with 0 ≤ P ≤ 1.

Case 1

The Patriacrat percentage of the total vote = C x D.

The Xenocrat percentage of the total vote = D + [(C x (1 – D)) x (1 – P)].

Then, we note that the Patriacrat percentage of the total vote < D for any values over the range of C and D, and the Xenocrat percentage of total vote ≥ D for any values over the range of C, D and P.

Then, we can see that the Xenocrat percentage always exceeds the Patriacrat percentage, and thus the Patriacrat policies never will be implemented — neither by majority nor by coalition government.

Thus, the policies of either the Plutocrats or Xenocrats will always be implemented and the ratio of Neomorphs to Paleomorphs will grow by 10% per year. The proportion of Neomorphs in the population will increase monotonically forever and eventually will approach 100% asymptotically, no matter how small the initial population of Neomorphs.

Case 2

Plutocrat policies are implemented.

The Plutocrat percentage of the total vote = C x (1 – SQRT(D)) x P.

Plutocrat policies will be implemented when C x (1 – SQRT(D)) x P ≥ 0.5, which is equivalent to P ≥ 0.5/ [(1 – D) x (1 – SQRT(D))].

If P < 0.5, then Plutocrat policies will never be implemented.

If P is barely above > 0.5, then Plutocrat policies will be implemented when D is very, very small.

As P grows above 0.5, Plutocrat policies will continue to be implemented as the population of Neomorphs grows slightly larger.

If P is very nearly 1.0 (or P = 1), then Plutocrat policies will be implemented when D is any amount up to approximately 0.1624.

From further analysis, it turns out that if P = 0.7382, then the Plutocrat policies will prevail as long as D is 0.0727 or smaller (thus allowing Neomorph growth up to this size). If P > 0.7382, then the cap on D grows (up to 0.1624 as P approaches 1.0). Once D crosses the threshold, Patriacrat policies prevail and Neomorph growth stops.

If P < 0.7382, then the Xenocrat policies step in as D crosses the threshold of being too large for the Plutocrats to gain a majority. The Xenocrat policies allow continued growth of the Neo population to a point, as analyzed in the following:

If the starting population of Neomorphs is relatively large and P > 0.643, then Xenocrat policies may always prevail.

Case 3

If conditions for Plutocrat policies to be implemented are not met, then Xenocrat policies will be implemented when the Xenocrat percentage of the total vote exceeds the Patriacrat percentage of the total vote, namely when:

D + [C x (1 – SQRT(D))] x (1 – P) > C x SQRT(D), which is equivalent to

P < 1 – [2 x (1 – D) x SQRT(D)]/[(1 – D) x (1 – SQRT(D))].

If P < 0.643 (approximately), this condition is met for all D (0 < D < 1). Thus, when P < 0.643, the policies of Patriacrats never will be implemented, regardless of the magnitude of D, and the ratio of Neomorphs to Paleomorphs will grow by 10% per year. The proportion of Neomorphs in the population will increase monotonically, forever, and will asymptotically approach 100%, no matter how small the initial population of Neomorphs.

If P = 0.644 (approximately), the condition is met for D < 0.18 (approximately). In this case, assuming that the initial population of Neomorphs is less than 0.18 of the total population, the proportion of Neomorphs in the population will increase until it approaches 0.18, enjoy one more 10% increase under the policies implemented in that regime, and then experience no further growth.

If P increases above 0.644, the condition is met for smaller values of D. For example, if P = 0.7382 (approximately), the condition is met for D < 0.0727 (approximately). In this case, the proportion of Neomorphs in the population will increase until it approaches 0.0727, enjoy one more 10% increase under the policies implemented in that regime, and then experience no further growth. This point (P = 0.7382, D = 0.0727) also happens to be the boundary for satisfying the Plutocrat majority scenario addressed in the first section of Case 2. Thus, if P > 0.7382, the boundaries of D are dictated by the Plutocrat majority scenario.

Interestingly, if P ≥ 0.644, the condition for Xenocrat percentage being greater than Patriacrat percentage is also met for larger values of D. For example,

• If P = 0.644, the condition is met if D > 0.21 (approximately).
• If P = 0.7382 (approximately), the condition is met for D > 0.30 (approximately).
• If P = 1.00, the condition is met for D > 0.39.

In these cases, the Xenocrat policies will prevail on an ongoing basis, and the percentage of Neomorphs will grow (asymptotically to 100%) forever. However, since there is a gap of greater than 10% growth between the small values of D that satisfy the condition and these larger values, this scenario only is encountered if the starting value of D is larger than the upper portion of this boundary condition.

An interesting situation occurs when 0.6430 < P < 0.6435. For example, when P = 0.6432, the condition for Xenocrat percentage being greater than Patriacrat percentage is met for D < 0.189 and for D > 0.200. This gap is narrow enough that a one-year 10% growth in Neomorph population can bridge the gap, and then continue growing on the other side of the gap. But it won’t necessarily do so — it all depends on how close to 0.189 the porportion of Neomorphs is as it approaches 0.189. It might land in the gap and never grow again, or it might leap the gap and continue growing forever.

Note: I have not addressed cases exactly on the boundary where the Patriacrat and Xenocrat vote counts are tied and the Plutocrat vote count is less than 50%. We are not told what policies would be implemented in this case. I also have not addressed the effects of rounding the vote count to a whole number of voters in cases where we are near the boundary.

Solutions were also submitted by Clive Keatinge, Brad Rosin and Ethan Triplett.